More about this Area and Volume of a Pyramid Calculator

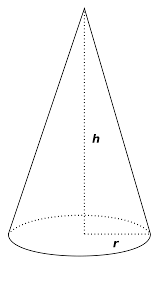
This calculator deals with square pyramids (those pyramids with squared base), for which you need to provided the side \(l\) of the base and the corresponding height \(h\). A square pyramid looks like:

How to Compute the Area and Volume of a Pyramid?

In order to compute the surface area and volume of square pyramid with side \(l\) and height \(h\) we use the following formulas:

\[ \text{Area} = l^2 + 2l\sqrt{\frac{l^2}{4} + h^2}\] \[ \text{Volume} = \frac{l^2 h}{3} \]

Computationally speaking, it is quite simple to compute the surface area and the volume of a square pyramid, by simply plugging the side \(l\) and height \(h\) in the above formulas. For example, if the side is \(l = 3\) and the height is \(h = 4\), we compute

\[ \text{Area} = l^2 + 2l\sqrt{\frac{l^2}{4} + h^2} = 3^2 + 2\cdot 3\sqrt{\frac{3^2}{4} + 4^2} = 34.632 \] \[ \text{Volume} = \frac{l^2 h}{3} = \frac{3^2\cdot 4}{3} = 12 \]

which completes the calculation.

Other geometric calculators

Our site provides a number of geometric calculators, including this sphere area and volume calculator , among many others.