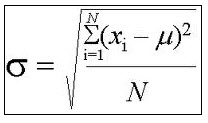Chi-Square Test of Independence
Instructions: This calculator conducts a Chi-Square test of independence. Please first indicate the number of columns and rows for the cross tabulation. Then type the table data, the significance level, and optionally the name of rows and columns, and the results of the Chi-Square test will be presented for you below:
More about the Chi-Square Test of Independence
Chi-Square of independence is a test used for categorical variables in order to assess the degree of association between two variables. Sometimes, a Chi-Square test of independence is referred as a Chi-Square test for homogeneity of variances, but they are mathematically equivalent. The idea of the test is to compare the sample information (the observed data), with the values that would be expected if the two variables were indeed independent. The main properties of a Chi-Square test of independence are:
- The distribution of the test statistic is the Chi-Square distribution, with \((r-1)\times(c-1)\) degrees of freedom, where r is the number of rows and c is the number of columns
- The Chi-Square distribution is one of the most important distributions in statistics, together with the normal distribution and the F-distribution
- The Chi-Square test of independence is right-tailed
The formula for a Chi-Square statistic is
\[\chi^2 = \sum_{i,j=1}^n \frac{(O_{ij}-E_{ij})^2 }{E_{ij} }\]One of the most common uses for this test is to assess whether two categorical variables are significantly related or not.
Usually the Chi-Square test for independence is referred as a 2-way crosstabulation test. If you have a one-way crosstabulation, you should use a Chi-Square test for goodness of fit .
What if you have paired data?
If you have paired data, instead of using the Chi-Square calculator, you should use this McNemar test calculator .